Trailing by 14 with just over 10 minutes remaining in regulation, quarterback Mark Piccirillo '19 helped orchestrate an incredible comeback as the Wesleyan University football team defeated Tufts University Sept. 23 in overtime, 24-17. The Saturday game was the second night game in school history, as the Cardinals picked up its first win of 2017.This is DiCenzo's eighth season coaching, and third season as head coach in 2017. Piccirillo found captain Eric Meyreles '18 in the corner of the end zone for a 24-yard score with :06 on the clock, and minutes later connected with senior Mike Breuler for a 14-yard…
(more…)
During the 13th annual Eat Local Challenge on Sept. 26, Wesleyan students, faculty and staff dined on a midday meal made entirely from local ingredients. The event challenged Bon Appétit Management Company staff to create a meal from products and ingredients harvested within a 150-mile radius of the campus. The meal included produce, meat, dessert and drinks from local farmers, ranchers, food crafters and fishermen. Food included house-smoked pork-belly bacon from Lucki 7 Livestock Co. in Rodman, N.Y.; Bloomsday cheese from Cato Corner in Colchester, Conn.; lobster, little-neck clams and mussels from Damariscotta, Maine; apple cider-glazed chicken and baked corn from…
At the 69th Annual Primetime Emmy Awards on Sept. 17, Sasha Alpert ’82, CSA, was awarded the Emmy for the Outstanding Casting for a Reality Program for A&E’s original docuseries Born This Way. The series follows seven young adults diagnosed with Down syndrome who pursue personal and professional success and try to defy expectations, according to A&E’s website. Born This Way earned a total of three Emmy wins, going into the evening with six nominations. It was the television show to receive the inaugural award for reality casting, a move that recognizes the process of casting an unscripted show. “I am…
Associate Professor of Economics Abigail Hornstein's article, "Words vs. actions: International variation in the propensity to fulfill investment pledges in China," was published in the journal China Economic Review in July 2017. Hornstein studied whether companies from certain countries were more likely than others to fulfill investment pledges. On average, she found that firms fulfilled about 59 percent of their pledges within two years. This number was lower for firms in countries with greater uncertainty avoidance, power distance, and egalitarianism; and higher for those in countries that are more traditional. She also found that popular attitudes toward China did not affect the likelihood…
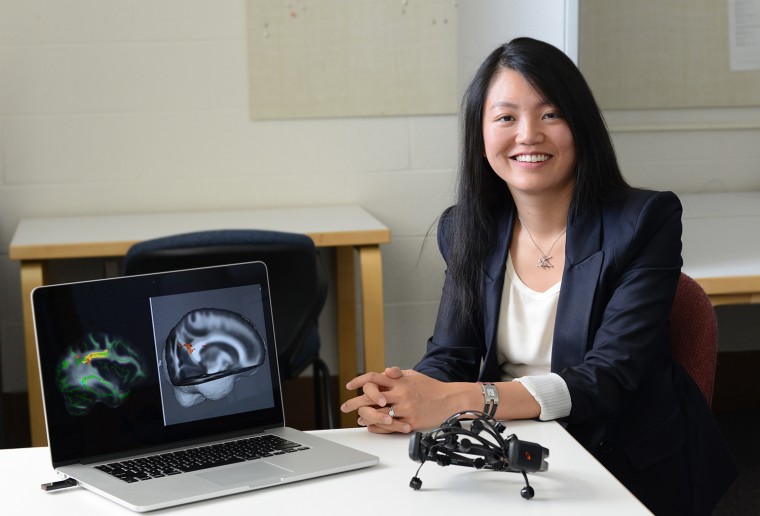
For her ongoing research in developing electromagnetic filters that block high power radiation, physics PhD candidate Eleana Makri recently received a Constantine and Patricia Mavroyannis scholarship from the Greek America Foundation. The $5,000 award will support her doctoral research during the 2017-18 academic year. At Wesleyan, Makri works with Professor Tsampikos Kottos in the development of the reflective limiter concepts that block high power radiation from damaging sensitive sensors, like the eye, while they allow low power radiation to reach the sensor for further processing. Kottos is professor of physics, professor of mathematics and professor of integrative sciences. The Mavroyannis scholarship…
Assistant Professor of History Victoria Smolkin was recently a guest on BBC Radio 4's "Beyond Belief" to discuss Soviet state atheism. Smolkin said that Lenin's conviction that banishing religion was necessary to create a revolutionary society was right ideologically, but wrong politically. "If they wanted to stay in power, they needed to accommodate religion, and they understood that," she said. "However, if they wanted to build a Communist society, ultimately religion had to go." (more…)
(By K Alshanetsky '17) Mika Reyes ’17 has stayed busy since graduating just last May, as both a summer fellow with the Horizons School of Technology and a year-long Product fellow with the Kleiner Perkins Caufield & Byers (KPCB) fellowship program. These prestigious programs have helped Reyes jump-start a career in tech. The Horizons Fellowship immerses university students looking to become leaders in technology in a rigorous summer program that teaches them how to build web and mobile applications and connects them with mentors in the field: startup founders, technology executives, and engineering leaders. Horizons requires no prior programming knowledge and chooses…
Psyche Loui, assistant professor of psychology, assistant professor of neuroscience and behavior, assistant professor of integrative sciences, is the author of a new publication on musical anhedonia—the lack of pleasure from music. Together with others in her lab, Loui studied an individual with musical anhedonia and compared his brain against a group of controls. They found that his auditory cortex was differently connected to his reward system, a finding which gives further support for the role of brain connectivity in the musical experience. The article, titled, "White Matter Correlates of Musical Anhedonia: Implications for Evolution of Music," was published Sept.…
On Sept. 22, the Wesleyan Student Assembly hosted its 27th annual Student Groups Fair on Andrus Field. The event provides an opportunity for students to meet with representatives of both new and established groups and network with university departments who provide annual programs. Wesleyan is home to more than 250 student organizations under the categories of activism, identity, sports, publications, visual arts, independent projects, the Office of Community Service and more. Groups include Wesleyan's pro-Israel political activism group, Cardinals for Israel; the Wesleyan Boxing Club; PINOY, the Filipino Student Association; the Basal Gang, a club for people interested in neuroscience…
Logan Dancey, assistant professor of government, and Jasmine Masand ‘15 are the co-authors of "Race and Representation on Twitter: Members of Congress’ Responses to the Deaths of Michael Brown and Eric Garner," published in Politics, Groups, and Identities in July 2017. This paper investigates the public responses of members of Congress to the deaths of Michael Brown and Eric Garner and the subsequent protests and grand jury decisions. To do so, the authors examined members’ engagement with the issue on Twitter, which became a platform for public protest with such hashtags as #BlackLivesMatter and #ICantBreathe. "We find that a member’s race is a…
Joseph Knee, the Beach Professor of Chemistry and Dean of the Natural Sciences and Mathematics Division, is the author of a new article published in the journal Physical Chemistry Chemical Physics (PCCP). This "Perspectives" article, which was commissioned by the PCCP editorial board and editorial office, is a high-profile look at work by Knee and his collaborators that has been going on for nearly a decade. Perspectives articles are intended to present an authoritative state-of-the-art account of a particular research field. The research by Knee and his collaborators, which is ongoing, uses experimental and computational methods to explore hydrogen bonding interactions, which are extremely important in the…